
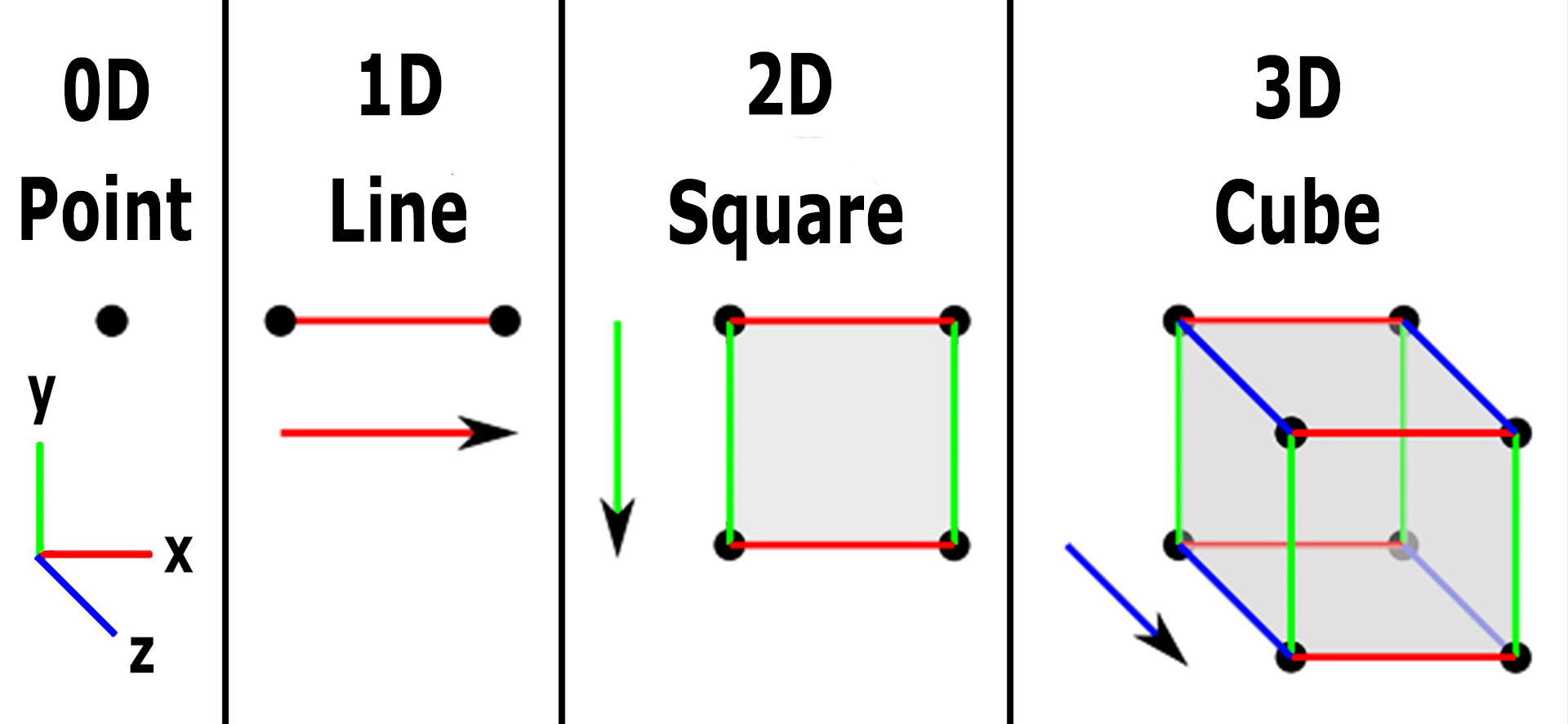
This procedure extends to all higher dimensions.

Of course we can continue this line of thought: 4-dimensional space, for a mathematician, is identified with the sets of quadruples of real numbers, such as (5,6,3,2). Just as a 2-dimensional plane can be described by pairs of coordinates such as (5,6) with reference to a pair of axes, so 3-dimensional space can be described by triples of numbers such as (5,6,3). Higher dimensions and hyperspheresĭo higher dimensions exist? Mathematics provides a surprisingly emphatic answer to this question. Over the last 50 years a subject called differential topology has grown up, and revealed just how alien these places are. Strange spheres really are the keys to higher dimensional worlds, and our understanding of them has increased greatly in recent years. But he could not have known how fortunate was the decision to represent Yog-Sothoth in this manner. Lovecraft had some interest in mathematics, and indeed used ideas such as hyperbolic geometry to lend extra strangeness to his stories (as Thomas Hull has discussed in Math Horizons). stupendous in its malign suggestiveness". Interestingly, on the rare occasions that Yog-Sothoth appears in the human realm, it takes the form of "a congeries of iridescent globes. In Lovecraft's mythology, the most terrible of these beings goes by the name of Yog-Sothoth. Lovecraft, these higher dimensions do indeed exist, and are home to all manner of evil creatures. Image by Claudio Rocchini, via Wikimedia Commons.Īccording to the early 20th century horror writer H.P. The curves are the projections of the hypersphere's parallels (red), meridians (blue) and so-called hypermeridians (green). This image comes from the projection of a 4-dimensional hypersphere. Just as a 3-dimensional object can be projected onto a 2-dimensional plane, so a 4-dimensional object can be projected onto 3-dimensional space.


 0 kommentar(er)
0 kommentar(er)
